img several http 答案 codeforce mes take 感謝 city

$ \Rightarrow $ 戳我進CF原題

G. Yet Another Maxflow Problem

---

time limit per test: 4 seconds memory limit per test: 256 megabytes input: standard input output: standard output

---

　

In this problem you will have to deal with a very special network.
　
The network consists of two parts: part $ A $ and part $ B $ .

Each part consists of n vertices; $ i $ -th vertex of part $ A $ is denoted as $ A_i $ , and $ i $ -th vertex of part $ B $ is denoted as $ B_i $ .
　
For each index $ i (1?≤?i?<?n) $ there is a directed edge from vertex $ A_i $ to vertex $ A_{i?+?1} $ ,
and from $ B_i $ to $ B_{i?+?1} $ , respectively.
Capacities of these edges are given in the input.
Also there might be several directed edges going from part $ A $ to part $ B $ (but never from $ B $ to $ A $ ).
　
You have to calculate the maximum flow value from $ A_1 $ to $ B_n $ in this network.
Capacities of edges connecting $ A_i $ to $ A_{i?+?1} $ might sometimes change,
and you also have to maintain the maximum flow value after these changes.
Apart from that, the network is fixed (there are no changes in part $ B $ ,
no changes of edges going from $ A $ to $ B $ , and no edge insertions or deletions).
　
Take a look at the example and the notes to understand the structure of the network better.
　

Input

The first line contains three integer numbers $ n, m $ and $ q (2?≤?n,?m?≤?2·10^5, 0?≤?q?≤?2·10^5) $
— the number of vertices in each part, the number of edges going from $ A $ to $ B $ and the number of changes, respectively.
　
Then $ n?-?1 $ lines follow, $ i $ -th line contains two integers $ x_i $ and $ y_i $ denoting
that the edge from $ A_i $ to $ A_{i?+?1} $ has capacity $ x_i $ and the edge from $ B_i $ to $ B_{i?+?1} $ has capacity $ y_i (1?≤?x_i,?y_i?≤?10^9) $ .
　
Then $ m $ lines follow, describing the edges from $ A $ to $ B $ .
Each line contains three integers $ x, y $ and $ z $ denoting an edge from $ A_x $ to $ B_y $ with capacity $ z (1?≤?x,?y?≤?n, 1?≤?z?≤?10^9) $ .
There might be multiple edges from $ A_x $ to $ B_y $ .
　
And then $ q $ lines follow, describing a sequence of changes to the network.
$ i $ -th line contains two integers $ v_i $ and $ w_i $ , denoting that the capacity of the edge from $ A_{v_i} $ to $ A_{v_i?+?1} $ is set to $ w_i (1?≤?vi?<?n, 1?≤?wi?≤?10^9) $ .
　

Output

Firstly, print the maximum flow value in the original network.
Then print $ q $ integers, $ i $ -th of them must be equal to the maximum flow value after $ i $ -th change.
　

Examples

input

 4 3 2
 1 2
 3 4
 5 6
 2 2 7
 1 4 8
 4 3 9
 1 100
 2 100

output

 9
 14
 14

　

Note

This is the original network in the example:

　

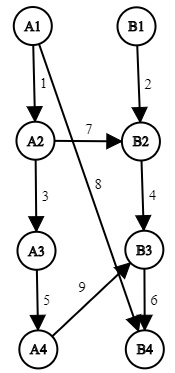
題目翻譯

• 一張圖分為兩部分，左右都有 $ n $ 個節點，

• $ A_i \rightarrow A_{i+1} $ 連邊， $ B_i \rightarrow B_{i+1} $ 連邊，容量給出

• 有 $ m $ 對 $ A_i \rightarrow B_j $ 有邊，容量給出

• 兩種操作

• 1.修改某條 $ A_i \rightarrow A_{i+1} $ 的邊的容量

• 2.詢問從 $ A_1 $ 到 $ B_n $ 的最大流

• $ 2 \le n,m \le 2 \times 10^5 $ 流量 $ \le 10^9 $

感謝@yybyyb 提供的翻譯
　

思路：

• 因為最大流的流量等於最小割的容量，所以最大流不好做的時候考慮最小割。

• 一些顯而易見的結論：

• 假如在 $ A $ 中，割掉 $ A_x $ 和 $ A_{x+1} $ 之間的有向邊，
  那麽割掉 $ A_y (y>x) $ 與 $ A_{y+1} $ 之間的有向邊是沒有意義的。

• 假如在 $ B $ 中，割掉 $ B_X $ 和 $ B_{x+1} $ 之間的有向邊，
  那麽割掉 $ B_y (y>x) $ 與 $ B_{y+1} $ 之間的有向邊是沒有意義的。

• 因此，在 $ A, B $ 中至多有一條邊屬於割集。

• 假設在 $ A $ 中被割掉的邊是 $ (A_x, A_{x+1}) $ ，在 $ B $ 中被割掉的邊是 $ (B_y, B_{y+1}) $ ，
  那麽任意 $ (A_u, B_v) (u \ge x $ 且 $ v> y) $ 都是屬於這個割集的。

• 因此，割的大小可以看作三部分：在 $ A $ 中的割集大小，在 $ B $ 中的割集大小，在 $ A, B $ 間的割集大小。

• 由於後兩部分不會改變（只改 $ A $ 的邊），考慮計算出它們。

• 考慮在 $ A $ 中從小到大枚舉 $ A_x $ ，表示割掉 $ A_x $ 和 $ A_{x+1} $ 之間的邊， $ x=n $ 表示不存在這條邊。

• 那麽下面要做的事情是在 $ B $ 中找到一個最優決策點 $ y $ ，使得後兩部分的和最小。

• 對於每加入一條 $ A, B $ 間的邊，對答案造成的影響是連續的一段，從 $ 1 $ 開始。

• 所以我們只需要寫一個支持區間加，求 $ [1, n] $ 的最小值的線段樹就好了。

• 合並兩部分的答案。這個可以用一個可刪堆或者線段樹來維護。

• 時間復雜度 $ O((n+m+q)\quad log_n ) $

codeforces CF903G Yet Another Maxflow Problem 線段樹